Description of lens and its different types with their applications

Related Terms of Lenses

Before discussing lens and their properties and application, one should be familiar with these terms.

Refractive Index

Refractive index is a ratio of the speed of light when it passes through the vacuum to its values when it passes through a specific medium.

\[n=c\hspace{0.1cm}v\hspace{3cm} (1)\]

where c is the speed of light in vacuum c = 3×10⁸ m/s, v is the speed of light in the medium and n is the refractive index value for that specific medium.

Law of Refraction

In optics, the law of refraction or Snell’s law has considerable importance. It defines the interaction of light when two or more transparent objects are placed, mounted, or joined with each other and how light behaves upon crossing the boundary dividing these objects, where each object has a different refractive index value. The path followed by light after crossing this boundary is given by the following equation, also known as Snell’s law, which was discovered by a Dutch Mathematician and astronomer in 1621.

\[n_1\sin\alpha_1=n_2\sin\alpha_2\hspace{3cm} (2)\]

n₁ and n₂ indicate the refractive indices values of two objects or media, and α₁ and α₂ represent the values of angles of incidence. Suppose light enters from a medium of low refractive index to a medium of high refractive index. In that case, the light slows down, for example, when light travels from air to glass medium. In other words, the light will bend towards the normal. And when light travels from an object of high refractive index to a medium of a low refractive index, it bends away from the normal.

Pole

It is the point situated precisely at the center where the surface meets the principal axis of reflecting the spherical surface of the lens.

Optical Centre

It is precisely the center of the lens where the principal axis crosses the midpoint of the lens.

Centre of Curvature

A lens consists of two spherical surfaces forming a part of the sphere. The center of curvature is exactly the center of these spheres.

Principal Axis

It is an imaginary line that passes through the pole of the lens and the center of curvature of the lens.

Aperture

The best-suited area of the lens where refraction of light is most likely to happen is called the aperture of the lens. The aperture of the lens is the effective diameter of its light-transmitting area.

Focus

The point at which parallel light rays, after passing through the principal axis, converge or diverge is the focus or principal focus of the lens. If the light is converged or diverged after passing parallel from the principal axis from the other side of the lens, then it will also have a principal focus on the opposite side of the lens. A lens usually has two principal focuses, f₁ and f₂.

Focal length

The distance between the focal point of the lens to the optical center of the lens is called the focal length. It is given as F or f of the lens and it is measured in units of length.

Power

The reciprocal of its focal length is called the power lens. The S.I unit of power is Diopter.

\[P=\frac{1}{f}\hspace{3cm} (3)\]

The power of the concave lens is always negative, while convex is positive.

What is a lens?/What are lenses?

Today, there are so many types of lenses. Most often, they are categorized as Simple or a Compound lenses. There is only a single magnifying material piece in a Simple lens, while the Compound lenses consist of aligned several simple lenses on a common axis in which they are placed. Sometimes there is an issue of optical aberration in simple lenses, but compound lenses do not have optical aberration, which is an advantage of using compound lenses. Compound lenses magnification is adjustable according to user requirements.

A lens is an optical device. It uses the law of refraction by which a light beam is transmitted, focused, and dispersed. It mainly comprises a small single transparent material that obeys the law of refraction. Lens has a curved body made up of glass or plastic. In the lens, a light beam is focused on a point, while in prism law of refraction is obeyed, but the light beam is not focused. The description of different lenses is given below.

1. Aspheric

Non-spherical lenses are also known as aspheric lenses. Their surface is not part of a sphere or a cylindrical shape. The design complexity of aspherical lenses helps to lessen the optical aberrations A much-reduced system size with better results with a single aspheric lens could be achieved rather than using a combination of simple lenses for the same purpose.

2. Cylinderical

Cylindrical lenses have their curvature on one axis. They focus light in line and convert elliptical light from the laser diode into a round beam. Examples of cylindrical lens are anamorphic lenses and motion pictures.

3. Fresnel

A Fresnel lens consists of an optical surface finely divided into narrow rings, resulting in a thinner lens and a lighter lens compared to other lenses.

4. Lenticular

These are a group of microlenses. They are often used in lenticular printing. These lenses produce images with an illusion of depths are also obtained with lenticular lenses.

5. Bifocal

A bifocal lens consists of two or more or graduated focal lengths.

6. Gradient Index

A gradient index lens has flat optical surfaces.

7. Axicon

Axicon lens contains a conical optical surface.

After discussing the major lenses and their design, we now discussion types of lenses.

Types of lens

There are mainly two types of the lens depending upon how light travels or bends when it passes through these lenses

Concave lens (diverging lens)
Convex lens (converging lens)

Concave lens (diverging lens)

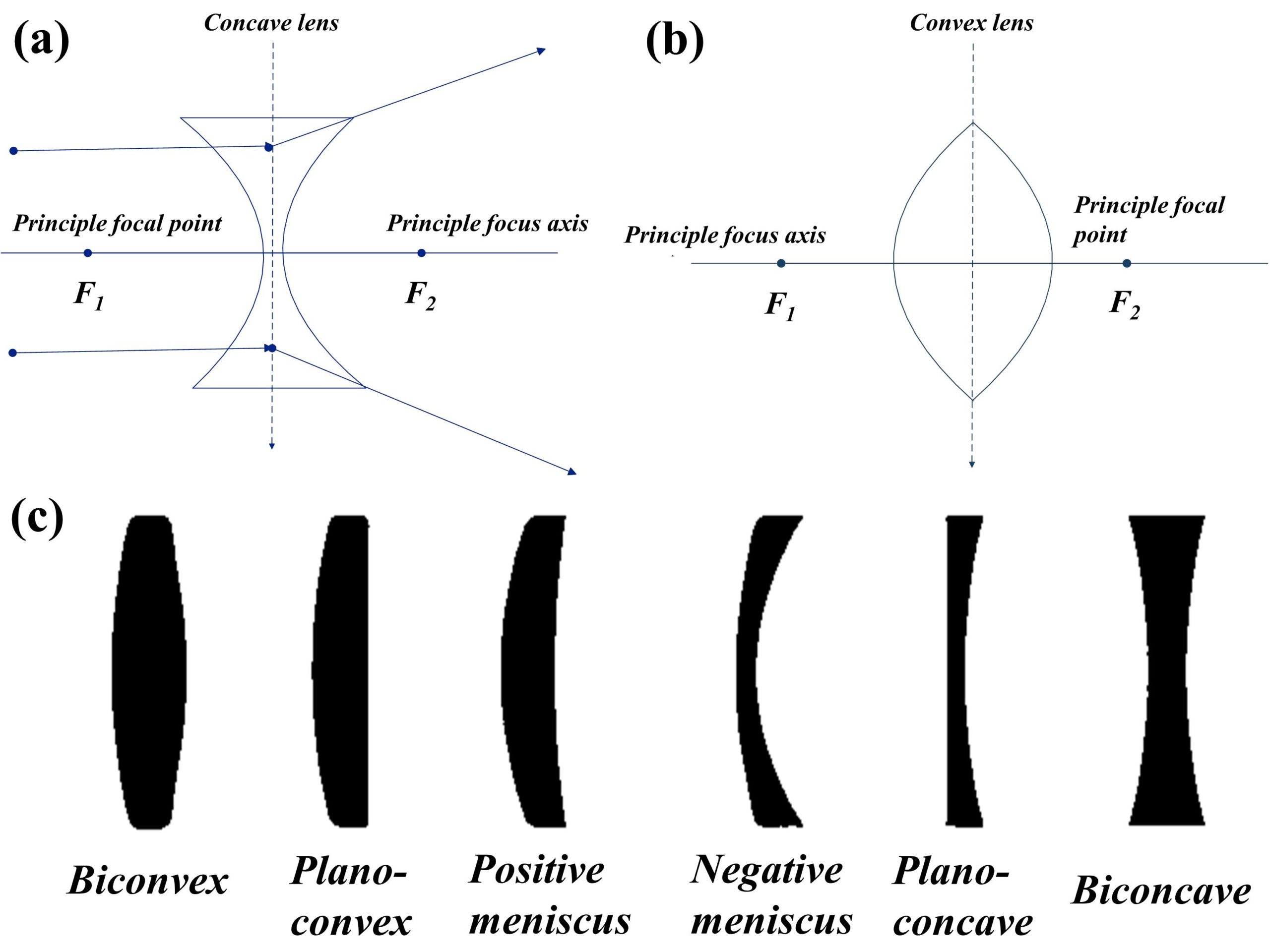
It is a lens that consists of at least one side, which is curved towards inwards. When light passes through a concave lens, it spreads it out, which is called divergence phenomena, and that is why concave lenses are also called diverging lenses. It has a thin center while it is thicker at both edges. It can form both real and virtual images, as shown in Fig.1(a).

Lens Formula

When an image is formed by a diverging lens (concave lens), to know the position of that image and the nature of that formed image, for example, if it is a real image or virtual image, a formula is derived.

\[\frac{1}{f}=\frac{1}{v}+\frac{1}{u}\hspace{3cm} (4)\]

This formula show f as the focal length of the lens, where u shows the object distance from the center of the lens. The term v shows the formed image distance from the center of the lens.

Similarly, the formula for concave lens magnification is given by Eq. 5.

\[M=\frac{h_i}{h_o}=\frac{v}{u}\hspace{3cm} (5)\]

The height of the image is expressed as h_i, and height of the object is expressed as h_o, and M is the magnification of the lens.

Types of concave lenses

There are three types of concave lenses, as shown in Fig. 1(c):

Biconcave lenses.
Plano concave lenses.
Convex-concave lenses (negative meniscus).

Bi-concave

Bi-concave lenses consist of a surface that is inwardly curved. Bi-concave lenses are being a type of concave lens their focal length is negative. Their design property is precisely the same curvature on both sides of lenses, making them symmetric. Because of symmetry, the unit distortion, coma, and conjugations are called out. Their usage includes increasing focal length and divergence of light.

Plano concave

A Plano concave lens is an optical lens it consists of a flat surface on one side and a concave (curved) surface on the other end. Its focal length is always negative. Beam expansion, light projection, or increased focal length of an optical system are the common uses of Plano concave lenses.

Convex-concave (negative meniscus)

Convexo-concave lenses consist of a side of a convex lens joined with a concave lens on the other side.

Applications of Concave lenses

They are used in glasses for having shortsightedness or myopia. A person suffering from myopic condition has a longer eyeball, and the image of an object present at a distance cannot reach the retina. Therefore, concave lenses are used to spread out the light before it comes to the eyeball. This helps a myopic person to see the distant object clearly. They are used in lasers CD /DVD drives, cameras, flashlights, and peepholes.

Convex lens (converging lens)

A convex lens is also called a converging lens which consists of a thick center and curved outwardly. They are mainly used to converge parallel rays of light coming from an object at a single spot or point, which is also called the focal point of the lens. In a convex lens, the thickness of the lens gradually decreases from the center to the side ends. The power of the convex lens is always positive. A convex lens is shown in Fig 1(b).

Types of Convex Lenses

There are three types of convex lenses, as shown in Fig. 1(c).

Plano-convex lens.
Double convex lens.
Concave-convex lens.

Plano-convex

A Plano-convex lens is an optical lens that consists of a flat surface on one side and a convex (curved) surface on another end. Its focal length is always positive. Beam convergence or increasing the focal length of an optical system are the common uses of Plano concave lenses.

Double Convex

A double convex lens consists of a curved surface on both sides. It is also called the bi-convex lens. The focal length of the double convex lens is shorter than the Plano-convex lens.

Concave-convex

Concave-convex lenses consist of a side made up of a convex lens joined with a concave lens on the other side.

Applications of Convex Lens

The prime example of the lenses is the lens found in the human eye. Their applications also include glasses that are used to correct long-sightedness or Hypermetropia. They are also used in high-performance cameras as they converge light and produce a clear image. Telescopes and microscopes use their magnifying properties to get a clear image. Optical devices mostly need longer focal lengths, which is why double convex lenses are commonly used. They are used in projectors and monoculars.

Image Formation by Lenses

There are three rules which are to be followed for image formation.

In the case of a convex lens, a ray of light passes parallel through the principal axis after the convex lens refracts it and then crosses the principle focus on the other side, as shown in Fig. 2(a). A ray of light diverges from the principal focus of the concave lens located on the same side of the lens, as shown in figure Fig. 2(b).
In the case of a convex lens, a ray of light crosses the principle focus after refracting through the lens. It then emerges precisely parallel to the principle axis, as shown in Fig. 2(c). In the case of a concave lens, a ray of light appears to be lying on the principal focus of the lens. After refracting through the concave lens comes out parallel to the principle axis, as shown in Fig. 2(d).
When a light ray passes exactly through the lens’s optical center, no deviations from its path of travel will be observed, as shown in Figs. 2(e-f).